The respected Comrade
"We should make it our major thrust to develop such core, basic technologies as IT, nanotechnology and bioengineering, along with such pivotal and beneficial scientific and technological fields as new materials and energy, space and nuclear technologies, and concentrate our efforts on them."
Ternary piezoelectric systems which consist of lead (plumbum) zirconate titanate (PZT) and the third composite oxide have been widely studied. In 1950s, researchers firstly developed PZT ceramics from the solid solution of PbTiO3 (ferroelectric, tetragonal) and PbZrO3 (antiferroelectric, orthorhombic). Since their discovery of piezoelectric properties in the binary PZT system, various solid solution ceramics composed of multi-component system including ternary composite PZT system (e.g. Pb(Mg1/3Nb2/3)O3-PZT, Pb(Yb1/2Nb1/2)O3-PZT, etc.) have been studied.
In ternary piezoelectric systems which consist of PZT and the third composite oxide, a triple point exists at the crossing point of boundaries between adjacent two phases. Usually, the third components – composite oxides are perovskite-type antiferroelectric or pseudo cubic phases. On crystallographical point of view, in pseudo cubic polycrystalline system, cubic lattices are deformed along the direction of face diagonals, forming 12 easy directions of polarizations which are equivalent to [100]-axis. So, there are 26 easy directions (e.g. tetragonal-6, rhombohedral-8 and orthorhombic-12) near the triple point of ternary polycrystalline ferroelectrics. And near the triple point tetragonal, rhombohedral and orthorhombic phases coexist and are phase-transformed by the external electric field, therefore the saturated polarization and deformation would be greater than those of single phase and near two phases boundary.
Recently, researchers of Institute of advanced science at
Inverse-pole-figure (IPF) model had been suggested to analyze domain-switching and deformation of single-phased polycrystalline ferroelectrics and then had been employed to analyze domain-switching and deformation near morphotropic phase boundary (MPB). Recently, IPF model was generalized to calculate saturated polarization near MPBs (including 3-phases MPB) analytically. And equilibrium composition was analytically evaluated by the generalized IPF model near tetragonal-rhombohedral (T-R) MPBs of polycrystalline ferroelectrics.
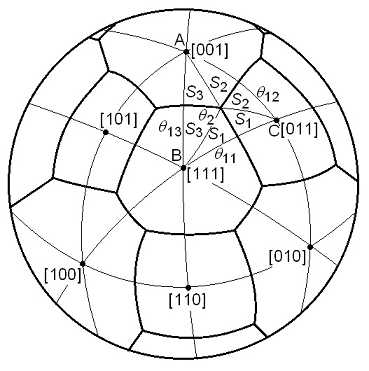
In this study, the numbers of crystalline particles involved in domain-switching near three phases (tetragonal-rhombohedral-orthorhombic, T-R-O) triple point have been calculated and domain switching which can bring out phase transformations have been considered by the generalized IPF model. According to the generalized IPF model, for T-R-O triple point, 26 easy axes are possibly pointed on the IPF spherical surface and therefore the IPF surface can be divided into 26 areas which have their centers on easy axes by circle arcs at same distances to adjacent two easy axes of polarization. Fig. 1 shows the divided IPF surface modeling domain switching near T-R-O triple point.
There exist 26 easy axes of polarization near the T-R-O triple point. Through polarization by electric field applied, crystalline particles can be involved in different phase transformations (e.g. T-R, T-O, R-T, R-O, O-T transformation).
In addition, phase equilibrium composition of the three phases coexisting near the T-R-O triple point has been evaluated from phase equilibrium condition that the number of crystalline particles involved in phase transformation from one phase to another is equal to the number of crystalline particles involved in inverse phase transformation. The phase equilibrium composition is determined as tetragonal phase ~27.54%, rhombohedral phase ~28.19% and orthorhombic phase ~44.27% by the numerical estimation based on the crystalline particle numbers involved in different phase transformations. Ideally, the phase equilibrium composition may be expected to minimize internal stress and change of intrinsic piezoelectric characteristics in T-R-O three-phase polycrystalline ferroelectrics.
Our results of this study were published in SCI journal entitled "Analytical study on the saturated polarization under electric field and phase equilibrium of three-phase polycrystalline ferroelectrics by using the generalized inverse-pole-figure model"(https://doi.org/10.1007/s11664-018-6249-y) in Journal "Journal of Electronic Materials" (2018, Vol. 47, No. 7, 3795-3799).